Answer:
First Case:
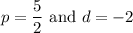
Second Case:
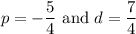
Explanation:
We know that the first three terms of an arithmetic series are:
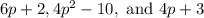
Since this is an arithmetic sequence, each subsequent term is d more than the previous term, where d is our common difference.
Therefore, we can write the second term as;
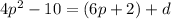
And, likewise, for the third term:

Let's solve for d for each of the equations.
Subtracting in the first equation yields:

And for the second equation:
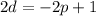
To avoid fractions, let's multiply the first equation by 2. Hence:

Therefore:
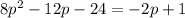
Simplifying yields:
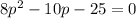
Solve for p. We can factor:

Factor:
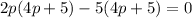
Grouping:
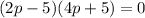
Zero Product Property:
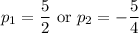
Then, we can use the second equation to solve for d. So:
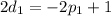
Substituting:
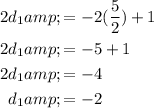
So, for the first case, p is 5/2 and d is -2.
Likewise, for the second case:
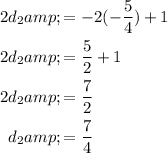
So, for the second case, p is -5/4, and d is 7/4.
By using the values, we can determine our series.
For Case 1, we will have:
17, 15, 13.
For Case 2, we will have:
-11/2, -15/4, -2.