Question

The length of the base of a rectangle is 6 less than 3 times the width. The perimeter of the rectangle is 52 inches. What is the length of the base?

Answer 1

The rectangle has a width of 18 inches and a height length of 8 inches.

Explanation:

The perimeter of a rectangle is described by the equation:

`p = 2w + 2l`

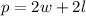
where l is width, h is height, and p is perimeter.

We're also told that the total perimeter is 52 inches.

We're also told that the length is six inches less than three times the width. We can express that as
`w = 3l - 6`.

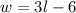We can plug that definition of w into the perimeter equation to find the length:

`p = 2w + 2l\\52 = 2(3l - 6) + 2 * l\\52 = 6l - 12 + 2l\\52 + 12 = 8l\\8l = 64\\l = 64/8\\l = 8`

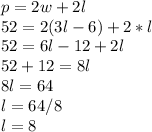
Now we can take that and the given perimeter, and substitute those into the perimeter equation to find the width:

`p = 2w + 2l\\52 = 2w + 2(8)\\52 = 2w + 16\\36 = 2w\\w = 36 / 2\\w = 18`

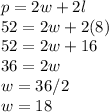
So the width is 18