Answer:
The system y=-3x + 4 and 3y = -9x+ 12 would contain infinitely many solutions.
Explanation:
Any system of equations that have the same equations would have infinitely many solutions.
In other words, if the two linear equations have the same slope and y-intercept, then the system of solutions would contain infinitely many solutions. So, their graphs would have exactly the same line
Also If the equation ends with a true statement, for instance, 3 = 3, then the system has infinitely many solutions or all real numbers.
Given the system of equations
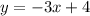

Writing both equations in the slope-intercept form

where m is the slope and b is the y-intercept
y=-3x + 4
Here,
m = -3
b = 4
3y = -9x+ 12
dividing both sides by 3
y = -3x + 4
Here,
m = -3
b = 4
As both equations have the same slope and y-intercept 'b'. Thus, the system of equations has infinitely many solutions.
Now checking:

substitute y = -3x+4
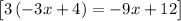
for y = -3x+4
Expressing y in terms of x
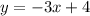
Thus, the solution would contain
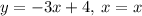
We know that If the equation ends with a true statement, for instance, x = x, then the system has infinitely many solutions or all real numbers.
Thus, the system y=-3x + 4 and 3y = -9x+ 12 would contain infinitely many solutions.