Question

A hypothesis will be used to test that a population mean equals 5 against the alternative that the population mean is less than 5 with unknown variance. We take a sample of size 23 from this population. What is the critical value for the test statistic for the significance level 0.01?

Answer 1

-2.508

Explanation:

From the given information:

The null hypothesis and the alternative hypothesis can be computed as:

`H_o : \mu = 5`

`H_1: \mu < 5`

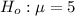
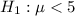
This is a left-tailed test.

The sample size n = 23

Then, the degree of freedom df = n - 1

df = 23 - 1

df = 22

The level of significance ∝ = 0.01

Using the student t-table to determine the critical value;

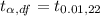`t_(\alpha, df) = t_(0.01, 22)` = -2.508 (since it is left tailed)