Question

Write a function in any form that would match the graph shown below.

Answer 1

f(x) = -2(x + 5)²(x - 2)²

Explanation:

Let the function shown by the graph is,

f(x) =
`k(x-a)^(p)(x-b)^q`

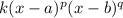
Here, x = a and b are the zeros of the function

p and q are the multiplicity of the function.

If the graph crosses x-axis at a zero x = a, p (multiplicity) will be odd.

If the graph just touches the x-axis at x = b, q will be even.

From the graph attached,

Zeros → x = -5, 2

Graph touches the x-axis, representing the even multiplicity.

Therefore, function will be,

f(x) = k(x + 5)²(x - 2)²

y-intercept from the graph → (0, -200)

From the given function,

-200 = k(0 + 5)²(0 - 2)²

-200 = 100k

k = -2

Therefore, function represented by the graph is,

f(x) = -2(x + 5)²(x - 2)²