Question

HERE IS A RECTANGLE ABCD 30CM=WIDTH AND 20CM=LENGTH AND LETTERS ABCD THE LENGH OF THE RECTANGLE IS INCREASED BY 10% AND THE WIDTH OF THE RECTANGLE I INCREASED BY 5%. WHAT IS THE PERCENTAGE INCREASE THE PERIMETER OF THE RECTANGLE?

Answer 1

7%

Explanation:

Perimeter of a rectangle

P = 2(w + l)

where:

• P = perimeter
• w = width
• l = length

Given:

• Width of rectangle = 30 cm
• Length of rectangle = 20 cm

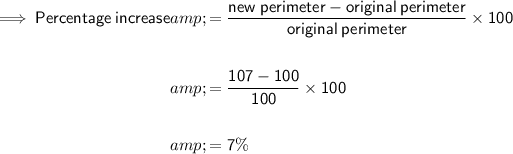
Therefore, the perimeter of the original rectangle is:

⇒ P = 2(30 + 20)

⇒ P = 2(50)

⇒ P = 100 cm

If the width is increased by 5%:

⇒ new width = 30 × 1.05 = 31.5 cm

If the length is increased by 10%:

⇒ new length = 20 × 1.1 = 22 cm

Therefore, the new perimeter will be:

⇒ P = 2(31.5 + 22)

⇒ P = 2(53.5)

⇒ P = 107 cm

Percentage Increase

`\sf PI=(final\:value-initial\:value)/(initial\:value) * 100`

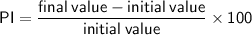
Substitute the values:

`\begin{aligned} \implies \sf Percentage\:increase & = \sf (new\:perimeter-original\:perimeter)/(original\:perimeter) * 100\\\\& = \sf (107-100)/(100) * 100\\\\& = \sf 7\%\end{aligned}`