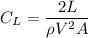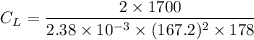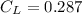Question

A Piper Cub airplane has a gross weight of 1700 lb, a cruising speed of 114 mph, and a wing area of 178 ft^2. Determine the lift coefficient of this airplane for these conditions.

Answer 1

As the plane is flying in the air, the force of weight is pulling the plane down. This force must be equal to the force of lift which is generated from the plane's translation true the air. Therefore, the next condition must be satisfied.

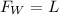`$F_W= L $` ................. (i)

The lift force of the plane is given by :

`$L=C_V (\rho)/(2) V^2 A$`

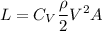
Since the weight force of the plane is given, from the condition (i), we also know the intensity of the lift force.

Therefore, we transform the lift force expression to derive the lift coefficient formula:

`$C_L=(2L)/(\rho V^2 A)$`

`$C_L=(2 * 1700)/(2.38 * 10^(-3 )* (167.2)^2 * 178) $`

`$C_L = 0.287$`