Answer:
1. t = 5.89 s
2. h = 170 m
3. Vf = 57.8 m/s
Step-by-step explanation:
1.
First, we analyze the horizontal motion of the golf ball. Assuming the air friction to be negligible, the horizontal motion will be uniform. So, e can use the following equation:
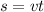
where,
s = horizontal distance covered by the golf ball = 471 m
v = horizontal speed of golf ball = 80 m/s
t = time taken by the golf ball in air = ?
Therefore,
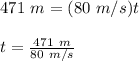
t = 5.89 s
2.
Now, we analyze the vertical motion. Using 2nd equation of motion:
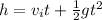
where,
h = height of cliff = ?
vi = vertical component of initial speed of ball = 0 m/s(ball was shot horizontally)
g = acceleration due to gravity = 9.81 m/s²
t = time of flight = 5.89 s
Therefore,
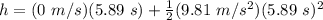
h = 170 m
3.
Now, we can use 1st equation of motion:

Vf = 57.8 m/s