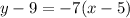Question

Write the equation in POINT-SLOPE FORM of the line that passes throught (5,9) and has a slope of -7.

Answer 1

The equation of line with slope -7 passing through (5,9) in point-slope form is:

`y-9 = -7(x-5)`

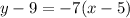
Explanation:

Given

Slope of line = m = -7

Point = (5,9)

The point slope form of equation is obtained from a point and slope. It is given by the equation:

`y-y_1 = m(x-x_1)`

We know that (x1,y1) = (5,9)

Putting the values we get

`y-9 = -7(x-5)`

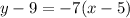
Hence,

The equation of line with slope -7 passing through (5,9) in point-slope form is:

`y-9 = -7(x-5)`