Question

On an air track, a 4-kg cart moving at 3 m/s collides with a 2-kg cart that is

initially at rest. If the two carts stick together, what will be their velocity
after the collision? Show all calculations, including any equation(s) used,
and include correct measurement units. *
Your answer

Answer 1

v₃ = 2 [m/s]

Step-by-step explanation:

To solve such problems we must use the principle of conservation of momentum. That is, the linear momentum is conserved before and after the collision.

P = m*v

where:

P = linear momentum [kg*m/s]

m = mass [kg]

v = velocity [m/s]

The momentum is conserved before and after the collision, in this way we can obtain the following equation.

`(m_(1)*v_(1))+(m_(2)*v_(2))=(m_(1)+m_(2))*v_(3)`

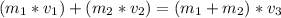
where:

m₁ = mass of the cart moving = 4 [kg]

v₁ = velocity of the cart moving before the collision = 3 [m/s]

m₂ = mass of the cart initially at rest = 2 [kg]

v₂ = velocity of the cart at rest = 0

v₃ = velocity of the two carts combined (carts stick together) after the collision [m/s]

`(4*3)+(2*0)=(4+2)*v_(3)\\v_(3)=12/6\\v_(3)=2[m/s]`