Answer:
(A) 95
(B) h=4
(C) 47, represents 0 hours of study
(D) h=9.63
(E) 92
Explanation:
(A) They are asking for the maximum of the graph of the equation which can be found using the vertex formula:
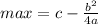
where 'a' is the coefficient of the first term, 'b' is the coefficient of the second term and 'c' is the third term.
So we have:
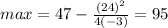
(B) To find the hours of study required to get 95 we need to set the equation equal to 95:
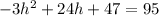
which can be rewritten as:

or
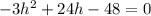
When we solve for 'h' we get h = 4
Therefore, studying 4 hours gives us a grade of 95, which is the maximum score from the equation.
(C) The function G(h) is our y and h is our x values. To find the y intercept, x (which is h in the equation) must be set to 0 and solved for y. This is because the y intercept is at the point where x =0.
So we have:

This is when h=0 and therefore is represents the grade when 0 hours of studying is done.
(D) To find how many hours of study can still produce a grade of 0, we need to set the function equal to 0 and solve for h.
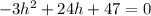
This gives us two results when solved quadratically:
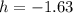 and
and

Obviously, time cannot be negative so the answer is 9.63 hours.
(E) If we study for 5 hours, h should be equal to 5.
So substituting 5 for h we get:
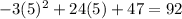
So 5 hours of study affords us a grade of 92.
Hope that explains it.