Question

How do you factor 9-6x+x^2

Answer 1

Explanation:

rewrite 9-6x+
`x^(2)` as
`x^(2)`-6x+9. (this step isn't necessary, but it's easier when the bigger term is in front)

There's two ways: using the quadratic formula, or just doing it in your head (for simple ones)

For the simple way:

think about what numbers not only multiply to equal c (represented by 9 in this case) but also add to equal -6 (represents b in this problem). There are two numbers for this problem that work: -3 and -3. So you would write the factored form as (x-3)(x-3)

Quadratic formula:

The formula is
`(-b)/(2a)` ±
`\frac{\sqrt{b^(2)-4ac } }{2a}`

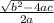
This formula works for equations in the form of a
`x^(2)`+bx+c.

Substitute in the values to get:

`(6)/(2) +\frac{\sqrt{-6^(2) -4(1)(9)} }{2}`

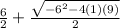
simplify:

3±
`(√(36-36) )/(2)`

3±
`(0)/(2)`

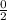
the answer is 3, which is the x-intercept. Write that as (x-3)(x-3)