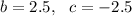Question

NO LINKS!!!
Help me with this problem​

Answer 1

c) b = 2.5, c = -2.5

Explanation:

Given system of equations:

`\begin{cases}3b-45c=120\\b-3c=10\end{cases}`

Rearrange the second equation to make b the subject:

`\implies b=10+3c`

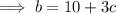
Substitute this into the first equation and solve for c:

`\implies 3(10+3c)-45c=120`

`\implies 30+9c-45c=120`

`\implies 30-36c=120`

`\implies -36c=90`

`\implies c=-2.5`

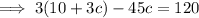
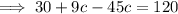
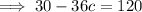
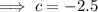
Substitute the found value of c into the rearranged second equation and solve for b:

`\implies b=10+3(-2.5)`

`\implies b=10-7.5`

`\implies b=2.5`

Therefore, the solution to the system of equations is:

`b = 2.5, \:\:\:c = -2.5`

Answer 2

Answer: b = 2.5, c = -2.5

Equation's:

1. 3b - 45c = 120
2. b - 3c = 10

Make b the subject:

3b - 45c = 120

3b = 120 + 45c

b = (120+ 45c)/3

= 40 + 15c ____ equation 1

`\rule{100}{1}`

b - 3c = 10

b = 10 + 3c ____ equation 2

Solve them Simultaneously:

b = b

10 + 3c = 40 + 15c

3c - 15c = 40 - 10

-12c = 30

c = -2.5

For b: 10 + 3c = 10 + 3(-2.5) = 2.5