Answer:
The functions are defined as:
- f(3) = -6
- f(0) = 3
- f(1) = 0
Explanation:
We are given a function:
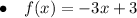
We need to find the values of the function at:
When we replace the x in f(x) with a number, we are saying that "f of 3" or "f of 0" or "f of 1." This means that when we change the x in f(x), we need to also change the rest of the x-variables in the function.
Therefore, to solve f(3), every x must be changed to 3.
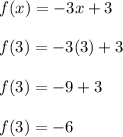
We can follow the same method for f(0) and f(1).
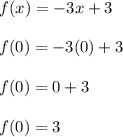
And finally, we can solve for f(1).
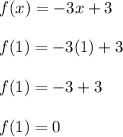
Therefore, the function is defined at each value as:
- f(3) = -6
- f(0) = 3
- f(1) = 0