Question

A company owns two dealerships, both of which sell cars and trucks. The first dealership sells a total of 164 cars and trucks. The second dealership sells twice as many cars and half as many trucks as the first dealership, and sells a total of 229 cars and trucks. The system of equations below represents this situation. {x+y=1642x+12y=229 How many cars does the first dealership sell?

Answer 1

98 cars

Explanation:

Given that:

The first dealership sells

x + y = 164 ------ (1)

The second dealership sells

2x +
`(1)/(2)`y = 229 --------(2)

How many cars does the first dealership sell?

Let determine the value of x and y to estimate that.

SO,

x + y = 164 ------ (1)

2x +
`(1)/(2)`y = 229 --------(2)

From equation (1)

x = 164 - y

Replacing the value of x = 164 - y into equation (2); we have:

`2(164-y) + (1)/(2)y = 229`

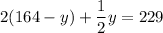
328 - 2y +
`(1)/(2)`y = 229

328 - 229 = 2y -
`(1)/(2)`y

`99 = (3)/(2)y`

`y = (99* 2)/(3)`

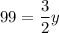
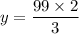
y = 66

Substitute y = 66 into equation (1) to find x

So;

x + y = 164

x + 66 = 164

x = 164 -66

x = 98

SO, if x = cars and y = truncks

The number of cars the first dealership sold = x = 98 cars