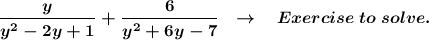
Factor y² - 2y + 1. Factor y² + 6y -7.
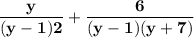
To add or subtract expressions, expand them so their denominators are the same. The least common multiple of (y - 1)² and (y - 1)(y + 2) it is (x + y)(y - 1)². Multiply
 by
by
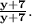 Multiply
Multiply
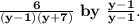
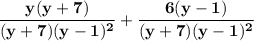
Since
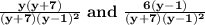 have the same denominator, add their numerators to add them together.
have the same denominator, add their numerators to add them together.

Do the multiplications on y(y + 7) + 6(y - 1).

Combine like terms in y² + 7y + 6y - 6.
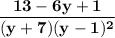
xpande (y+7)(y−1)².

