Answer:
The least number of items to produce is 41
Explanation:
Average Cost
Given C(x) as the cost function to produce x items. The average cost is:
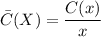
The cost function is:

And the average cost function is:
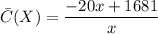
We are required to find the least number of items that can be produced so the average cost is less or equal to $21.
We set the inequality:
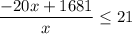
Multiplying by x:
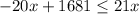
Note we multiplied by x and did not flip the inequality sign because its value cannot be negative.
Adding 20x:
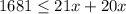

Swapping sides and changing the sign:

Dividing by 41:

The least number of items to produce is 41