Answer:
P(X > 10) = 0.0228
P(X< 5.5) = 0.1587
Explanation:
Given that:
Mean = 7.0
standard deviation = 1.5
To find:
a) P(X>10)
Using the standard normal variation
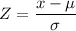
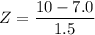
Z =2.0
So;
P(X > 10) = P(Z > 2.0)
P(X > 10) = 1 - P(Z < 2.0)
From the tables
P(X > 10) = 1 - 0.9772
P(X > 10) = 0.0228
b) To find:
P(X < 5.5)
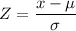
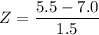
Z = -1.0
However;
P(X< 5.5) = P(Z < -1.0)
P(X< 5.5) = 0.1587