Question

Write the linear function f with the values f(1) = 1 and f(-3) = 17. (Note: Use f(x) instead of y.) Show work to get full credits.

Answer 1

Given:

f(1) = 1 and f(-3) = 17

To find:

The lines function f.

Solution:

If f(a) = b, it means the function f passes through (a,b).

Here, f(1) = 1, it means the function f passes through (1,1).

f(-3) = 17, it means the function f passes through (-3,17).

If a linear function passes through two point
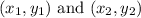`(x_1,y_1)\text{ and }(x_2,y_2)`, then the equation of line is

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

The linear function f passes through (1,1) amd (-3,17). So, the equation of linear function is

`y-1=(17-1)/(-3-1)(x-1)`

`y-1=(16)/(-4)(x-1)`

`y-1=-4(x-1)`

`y-1=-4x+4`

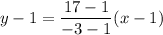
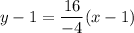
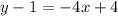
Add 1 on both sides.

`y-1+1=-4x+4+1`

`y=-4x+5`

Put y=f(x), to write in function notation.

`f(x)=-4x+5`

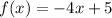
Therefore, required lines function is
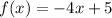`f(x)=-4x+5`.