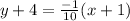Question

Write the equation of the line that passes through the points (-1,-4) and (9,-5).

Put your answer in fully reduced point-slope form, unless it is a vertical or horizontal
line.

Answer 1

The equation in point-slope form is:
`y+4=(-1)/(10)(x+1)`

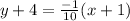
Explanation:

Write the equation of the line that passes through the points (-1,-4) and (9,-5).

The point slope form is:
`y-y_1=m(x-x_1)`

Where m is slope and x₁ and y₁ are the points given

Finding Slope

Slope can be found of given points using formula:
`Slope=(y_2-y_1)/(x_2-x_1)`

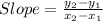
We have
`x_1=-1, y_1=-4, x_2=9, y_2=-5`

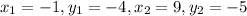
Putting values and finding slope

`Slope=(y_2-y_1)/(x_2-x_1)\\Slope=(-5-(-4))/(9-(-1))\\ Slope=(-5+4)/(9+1)\\ Slope=(-1)/(10)`

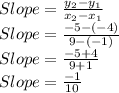
So, slope m = -1/10

Using point (-1,-4) and slope m = -1/10 the equation is:

`y-y_1=m(x-x_1)\\y-(-4)=(-1)/(10)(x-(-1))\\y+4=(-1)/(10)(x+1)`

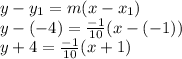
So, the equation in point-slope form is:
`y+4=(-1)/(10)(x+1)`