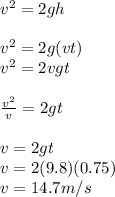Question

6. You are holding a rock with a mass of 0.30 kg. You let the rock drop from your hand. It hits the ground 0.75

seconds later. How fast was the rock moving right before it hit the ground? For credit, show work in detail.

Answer 1

v = 14.7m/s

Step-by-step explanation:

When the rock was at the top of cliff it had only Potential Energy and no Kinetic Energy but soon as the rock hit the ground all the Potential Energy converted into Kinetic Energy so by the Law of Conservation of Energy

`Potential\ Energy = Kinetic\ Energy\\mgh=(1)/(2)mv^2\\\\v^2=(2mgh)/(m) \\\\v^2=2gh`

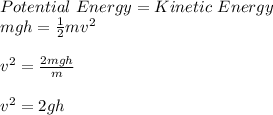
because all of that potential energy got converted into kinetic energy so we use the following formula as we need to calculate the velocity (v)

`Potential\ Energy = Kinetic\ Energy\\mgh=(1)/(2)mv^2\\\\v^2=(2mgh)/(m) \\\\v^2=2gh`

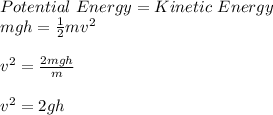
here we know
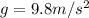`g = 9.8m/s^2` because the rock was under free fall motion so it had acceleration of 9.8 but we do not the height which is h but we do know the formula:

`Velocity=(Displacement)/(Time)`

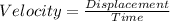
the height h is the displacement so we replace height with the following:

`Velocity=(Displacement)/(Time)\\\\Displacement=Velocity*Time\\h=vt`

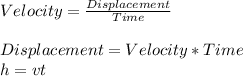
now, insert h = vt in the above equation

`v^2=2gh\\\\v^2=2g(vt)\\v^2=2vgt\\\\(v^2)/(v) =2gt\\\\v=2gt\\v=2(9.8)(0.75)\\v=14.7m/s`