Answer:
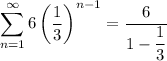
Explanation:
The given summation is infinite geometric series as it’s shown an exponent which geometric series/sequence has.
Let’s review partial geometric series formula:
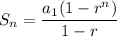
When we input the limit as n approaches infinity:
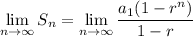
Consider each intervals.
( 1 ) When |r| > 1
Since |r| is greater than 1, it’s defined to approach infinity. Since it approaches infinity then we can say that the series diverges to infinity.
( 2 ) When r = 1
For r = 1, the equation for partial sum is S = na where a is first term and n is number of first term. For r = 1, the sum will continue to grow and will eventually approaches infinity. Henceforth, the series diverges.
( 3 ) When r ≤ -1
In this interval, the series oscillates which is defined to be divergent. Hence, the series diverges.
( 4 ) When |r| < 1
In this interval,
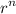 will approach 0 as accorded to exponential graph.
will approach 0 as accorded to exponential graph.
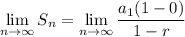
Hence, for |r| < 1, the geometric series will converge to:
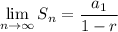
We can also write in summation notation form as:
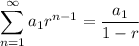
Therefore, from the given problem, it’s fortunately that the series is already in the same pattern as formula. Therefore, we simply apply the formula in.
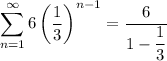
Therefore, the answer is third choice! Please let me know if you have any questions!