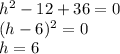Answer:
h=6
Explanation:
since
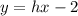 is an equation for a line which intersects with the curve
is an equation for a line which intersects with the curve
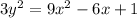 . The point of intersection, let's say
. The point of intersection, let's say
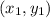 , should satisfy the two equations. As a result, the value of y in the second equation can be replaced with the value of y in the first equation as the following,
, should satisfy the two equations. As a result, the value of y in the second equation can be replaced with the value of y in the first equation as the following,
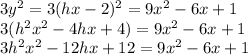
therefore, the latter equation can be rewritten in a quadratic equation form as the following,
 = 0
= 0
if the line is tangent to the curve, it means that the line touches the curve at one point, therefore the discernment of the second order equation will be equal to zero for the famous quadratic equation solution.

where
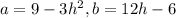 and
and
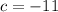 , as a result, the following equations can be deduced,
, as a result, the following equations can be deduced,
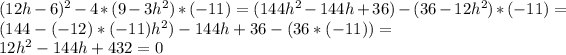
therefore, dividing both sides by 12