Answer:
Option A: (5,0)
Explanation:
Given
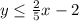
Required
Select an ordered pair
Option A: (5,0)
This means
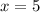

Substitute these values in
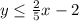

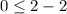
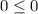
This is a true statement.
Option B: (0,5)
This means

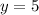
Substitute these values in
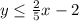
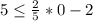
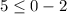
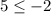
This is a false statement.
Option C: (-2,5)
This means

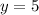
Substitute these values in
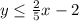


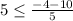
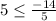
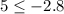
This is a false statement.
Option D: (-5,2)
This means

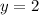
Substitute these values in
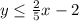

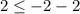

This is a false statement.
Only option A represents a true statement,
Hence, option A is a solution to the inequality