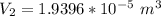Answer:
The value is
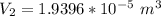
Step-by-step explanation:
From the question we are told that
The depth at which the bubble is released is

The volume of the air bubble is

Generally from the ideal gas law

Given that n , R , T are constant we have that
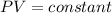
So
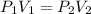
Here
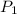 is the pressure of the bubble at the depth where it is released which i mathematically represented as
is the pressure of the bubble at the depth where it is released which i mathematically represented as
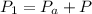
Here
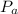 is the atmospheric pressure with value
is the atmospheric pressure with value
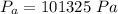
and
 is the pressure due to the depth which is mathematically represented as
is the pressure due to the depth which is mathematically represented as
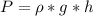
So
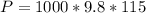
=>

Here
 is the density of pure water with value
is the density of pure water with value
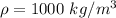
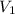 is the volume of the bubble at the depth where it is released
is the volume of the bubble at the depth where it is released
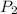 is the pressure of the bubble at the surface which is equivalent to the atmospheric temperature
is the pressure of the bubble at the surface which is equivalent to the atmospheric temperature
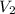 is the volume of the bubble at the surface
is the volume of the bubble at the surface
So
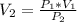
=>
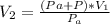
=>
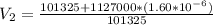
=>