Answer:
Number of arrangements is 604800
Explanation:
Given
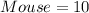 --- Total
--- Total
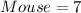 --- Arrangement
--- Arrangement
Required
Determine the number of ways
Since, it is an arrangement, then we make use of the following permutation formula to answer this question.
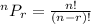
Where

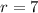
So:
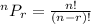
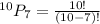

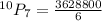
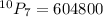
Hence, number of arrangements is 604800