Question

A smoke jumper jumps from a plane that is 1900 ft above the ground. The function h=-16t+1900 gives the​ jumper's height h in feet during the free fall at t seconds. a. How long is the jumper in free fall if the parachute opens at 1000​ ft? b. How long is the jumper in free fall if the parachute opens at ​ft? c. What is a reasonable domain and range for the function​ h? a. The jumper is in free fall for nothing s if the parachute opens at 1000 ft

Answer 1

a) The jumper opens the parachute in 7.5 seconds.

b) The jumper opens the parachute in approximately 7.706 seconds.

c) There are two possible choices for the function:

Case a):
`Dom\{h(t)\} = [0\,s,7.5\,s]`,
`Ran\{h(t)\}=[1000\,ft,1900\,ft]`

Case b):
`Dom\{h(t)\} = [0\,s,7.706\,s]`,
`Ran\{h(t)\}=[950\,ft,1900\,ft]`

Explanation:

Note: The equation presented in statement is incorrect. The correct formula for free fall is:

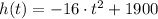`h(t)=-16\cdot t^(2)+1900` (1)

Where:

`t` - Time, measured in seconds.

`h` - Height above the ground, measured in feet.

a) If we know that
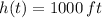`h(t) = 1000\,ft`, then the final instant of the free fall stage is:

`-16\cdot t^(2)+1900 = 1000`

`16\cdot t^(2) = 900`

`t = 7.5\,s`

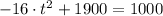
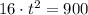
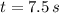
The jumper opens the parachute in 7.5 seconds.

b) How long is the jumper in free fall if the parachute opens at 950 ​ft?

If we know that
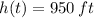`h(t) = 950\,ft`, then the final instant of the free fall stage is:

`-16\cdot t^(2)+1900 = 950`

`16\cdot t^(2) = 950`

`t \approx 7.706\,s`

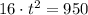
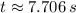
The jumper opens the parachute in approximately 7.706 seconds.

c) There are two possible choices for the function:

Case a):
`Dom\{h(t)\} = [0\,s,7.5\,s]`,
`Ran\{h(t)\}=[1000\,ft,1900\,ft]`

Case b):
`Dom\{h(t)\} = [0\,s,7.706\,s]`,
`Ran\{h(t)\}=[950\,ft,1900\,ft]`