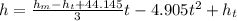Question

Jeff is standing on top of a tree next to a mysterious monolith (a big rectangular box). At time t = 0, Jeff threw a coconut vertically upwards. It landed on the top of the monolith 3 seconds later. The height of the coconut above the ground after t seconds is

Answer 1

`h=(h_(m)-h_(t)+44.145)/(3)t-4.905t^(2)+h_(t)`

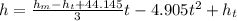
(if the heights are measured in meters)

Explanation:

In order to solve this problem we will need to first draw a diagram of the situation (see attached picture) and then, make use of the following physics equation:

`y_(f)=(1)/(2)at^(2)+v_(0)t+y_(0)`

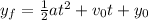
where:

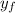`y_(f)`=height of the object at a given time t

a=acceleration due to gravity

t=time

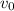`v_(0)`=initial velocity of the object

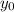`y_(0)`=initial height of the object.

we know most of this data, except for the height of theh object at the given time t and the initial velocity of theh object. We can make use of the given time to write the velocity in terms of the height of thte tree and the height of the monolith.

So let's substitute the values we know so far:

`h=(1)/(2)(-9.81)t^(2)+v_(0)t+h_(tree)`

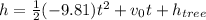
the problem tells us that after 3 seconds, the object reaches the height of the monolith, so let's do the substitution:

`h_(monolith)=(1)/(2)(-9.81)(3)^(2)+v_(0)(3)+h_(tree)`

we can now solve this equation for the initial velocity, so we get:

`h_(monolith)-h_(tree)=-44.145+3v_(0)`

`h_(monolith)-h_(tree)+44.145=3v_(0)`

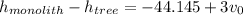
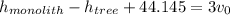
so:

`v_(0)=(h_(monolith)-h_(tree)+44.145)/(3)`

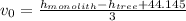
and now we can substitute this back into the original formula to get the equation that represents the height of the coconut above the ground after t seconds:

`h=(h_(m)-h_(t)+44.145)/(3)t-4.905t^(2)+h_(t)`