Question

Find the length of the third side to the nearest tenth.
8
5

Answer 1

The length of third side is: 6.3

Explanation:

Given triangle is a right triangle where

Base = ?

Perpendicular = 5

Hypotenuse = 8

Let x be the base

As it is a right-angled triangle, Pythagoras theorem can be used to find the third side

`Hypotenuse^2 = Base^2 + Perpendicular^2`

Putting the known values

`(8)^2 = x^2 + (5)^2\\x^2 = (8)^2-(5)^2\\x^2 = 64-25\\x^2 = 39\\`

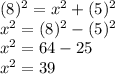
Taking square root on both sides

`√(x^2) = √(39)\\x = 6.24499`

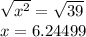
Rounding off to the nearest 10th

Base = 6.3

Hence,

The length of third side is: 6.3