Question

For each question, solve for the length of the unknown side, as

denoted by the variable. You may round your answers to the nearest
tenth, if necessary. Make sure you show all steps needed to solve the
problem.

Answer 1

a = 9

Explanation:

lets make is short and simple

Pythagorean theorem: a² + b² = c²

a² + 12² = 15²

a² = 15² - 12²

a = √81

a = 9

Answer 2

`a=9`

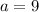
Explanation:

In this problem, you can use the Pythagorean Theorem (
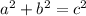`a^(2) +b^(2) =c^(2)`) to find what a is. The steps are shown below:

Steps (variables a, b, and c are in italics):

-You can rearrange the formula from the Pythagorean Theorem, which is
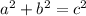`a^(2) +b^(2) =c^(2)` to only get a because we want to know what a is.

Rearranged:
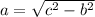`a=\sqrt{c^(2)-b^(2) }` or
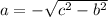`a=-\sqrt{c^(2)-b^(2) }` It has to ways/options for a because when finding the square root of a number, it can be either positive or negative. But in this problem, it’s going to be positive because we’re finding the length of something.

-Since we know what c and b are, you can plug them into the new formula:

`a=\sqrt{15^(2)-12^(2) }`

-Simplify it:

`a=√(225-144)`

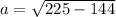
-Subtract inside the radical:

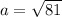`a=√(81)` Then simplify it to get
`a=9`.

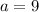You can check your answer by putting a back into the original Pythagorean Theorem formula (
`a^(2) +b^(2) =c^(2)`):

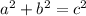Steps

-Plug a, b, and c into the formula
`a^(2) +b^(2) =c^(2)`:

`(9)^(2) +(12)^(2) =(15)^(2)`

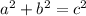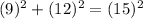
-Simplify to get:

`81+144=225`

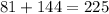
-Add:

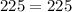`225=225` This is very true. So our answer is correct.

ANSWER:
`a=9`

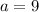
Sorry for the long explanation, but I hope you understand and that this helps with your question! :)