Question

In a popular online role playing game, players can create detailed designs for their character's "costumes," or appearance. Scarlett sets up a website where players can buy and sell these costumes online. Information about the number of people who visited the website and the number of costumes purchased in a single day is listed below. 236 visitors purchased no costume. 202 visitors purchased exactly one costume. 40 visitors purchased more than one costume. Based on these results, express the probability that the next person will purchase no more than one costume as a fraction in simplest form.

Answer 1

The probability that the next person will purchase no more than one costume
`(219)/(239)`.

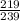Explanation:

The data for a single day is s follows:

• 236 visitors purchased no costume.
• 202 visitors purchased exactly one costume.
• 40 visitors purchased more than one costume.

The total number of customers is, N = 236 + 202 + 40 = 478.

Let X denote the number of costumes purchased by the next person.

Compute the probability that the next person will purchase no more than one costume as follows:

`P (X\leq 1)=1-P(X>1)`

`=1-(40)/(478)\\\\=(478-40)/(478)\\\\=(438)/(478)\\\\=(219)/(239)`

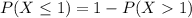
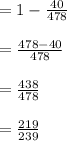
Thus, the probability that the next person will purchase no more than one costume
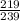`(219)/(239)`.