Question

A bus leaves a station traveling north at 70 kilometers per hour. Half an hour later a

second bus leaves the same station traveling north at 80 kilometers per hour. How far
from the station will the buses meet?

Answer 1

280 km

Explanation:

Given that a bus, say
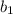`b_1`, leaves a station traveling north at speed of 70km/hour.

Half an hour later the second bus, say
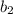`b_2`, leaves a station traveling north at speed of 70km/hour.

As, distance = speed x time,

so, the distance traveled by bus
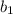`b_1` in 0.5 hours = 70 x 0.5 = 35 km.

Note that, both the buses are traveling in the same direction and when the second bus leaves the station, the first bus already covered a distance of 35 km.

Let the second bus took
`t` hours to meet the first bus and both the buses meet at a distance of
`d` km from the station.

The distance traveled by the first bus from the station,
`d = 35 + 70t` km

`\Rightarroe t= \frac {d-35}{70}\cdots(i)`

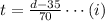
and the distance traveled by the second bus,
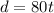`d = 80t` km

`\Rightarrow t=\frac {d}{80}\cdots(ii)`

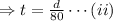
Now, equation the equation (i) and (ii), we have

`\frac {d-35}{70}=\frac {d}{80} \\\\\Rightarrow 8(d-35)=7d \\\\\Rightarrow 8d - 280 = 7d \\\\\Rightarrow 8d-7d=280 \\\\\Rightarrow d = 280 km`

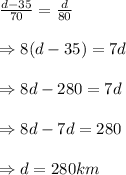
Hence, both the bus will meet at a distance of 280 km from the station.