Question

If ‘c’ is directly proportional to‘d’ and c = 24 when d = 4

Find an equation connecting ‘c’ and ‘d’

Find ‘c’ when d = 8

Find ‘d’ when c = 54

Answer 1

When d = 8

c = 48

when c = 54

d = 9

Explanation:

We have the equation c is proportional to d

`c \: \: \: \alpha \: \: d`

ie

`(c)/(d) = \: a \: constant`

here given that c = 24 and d = 4 so,

`(c)/(d) = (24)/(4) = 6`

so we have to find values for c and d which gives

c/d = 6

so when d = 8

`(c)/(8) = 6 \\ so \: \\ c = 8 * 6 = 48 \\ \\ and \: when \: \\ c \: = 54 \\ \\ (54)/(d ) = 6 \\ \\ d \: = (54)/(6) = 9`

Answer 2

• c = 6d
• c = 48 when d=8
• d = 9 when c=54

Explanation:

We know:

‘c’ is directly proportional to‘d’

and

c = 24 when d = 4

W can write this in mathematical form as:

c∝d

Removing the proportionality symbol, k is the constant of proportionality

c = kd

To find the value of k, putting the known values of c and d

`24 = k * 4\\k = (24)/(4) = 6`

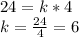
So the equation becomes

`c = 6d`

To find the value of c when d = 8

`c = 6*8\\c = 48`

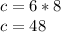
To find the value of d when c=54

`54 = 6d\\d = (54)/(6)\\d = 9`

Hence,

• c = 6d
• c = 48 when d=8
• d = 9 when c=54