Question

Measuring Current as a Function of Voltage with a 20 Q Resistor

Voltage
M
Current Calculated
(A)
Current Experimental
(A)
1
5
10
20
50
Measuring Current as a Function of Resistance at 25 V
Resistance
Current Calculated
(10)
(A)
10
20
100
200
Determining Current in a Parallel Circuit
Observed
Resistor Set
(0)
Total
Resistance
Calculated
Current
(A)
Current
(A)
(10)
20, 20, 20
20, 20, 200
Voltage needed to raise current to 3.75 A (20, 20, 200 resistor set):
Calculated
Observed:
Calculating Power of Circuit Components
Current through Each Bulb
(A)
Table B
Table C
Table D
Observed Total Current
(A)
Current Experimental
(A)
Observed Current
through Each Resistor
(A)
Power Usage per Bulb
(

Answer 1

Resistance at 25 V

Step-by-step explanation:

(10)

(A)

10

20

100

200

Determining Current in a Parallel Circuit

Observed

Resistor Set

(0)

Total

Resistance

Calculated

Current

(A)

Current

(A)

(10)

20, 20, 20

20, 20, 200

Voltage needed to raise current to 3.75 A (20, 20, 200 resistor set):

Calculated

Observed:

Calculating Power of Circuit Components

Current through Each Bulb

(A)

Table B

Table C

Table D

Observed Total Current

(A)

Current Experimental

(A)

Observed Current

through Each Resistor

(A)

Power Usage per Bulb

(

Answer 2

Plotting the graph between current (I) and potential difference (V) allows the determination of the resistance (R) of the resistor by analyzing the slope of the resulting straight line.

To determine the resistance (R) of the given resistor, we can plot a graph between current (I) and potential difference (V) using the provided data. According to Ohm's Law, the relationship between current, potential difference, and resistance is given by the equation V = IR.

Using the given data points (V, I), plot the current (I) on the y-axis and potential difference (V) on the x-axis. A linear relationship in the form of a straight line passing through the origin indicates a resistor with constant resistance.

After plotting the graph, the slope of the line represents the resistance (R) according to Ohm's Law
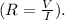`(\(R = (V)/(I)\)).` The reciprocal of the slope gives the resistance.

For a straight-line graph, the equation can be written as y = mx, where (y) is the dependent variable (I), m is the slope, and x is the independent variable (V).

In this case, the slope m is equal to R, the resistance of the resistor.

In summary, by plotting a graph between current (I) and potential difference (V), the resistance (R) of the resistor can be determined by finding the slope of the line, indicating the constant relationship between current and potential difference according to Ohm's Law.