Question

Help please i dont get it

Answer 1

`\\ \rm\Rrightarrow log_4x+log_4(x+6)=2`

`\\ \rm\Rrightarrow log_4(x(x+6))=2`

• Take 4 right

`\\ \rm\Rrightarrow x(x+6)=4^2`

`\\ \rm\Rrightarrow x^2+6x=16`

`\\ \rm\Rrightarrow x^2+6x-16=0`

`\\ \rm\Rrightarrow x^2-2x+8x-16=0`

`\\ \rm\Rrightarrow x(x-2)+8(x-2)=0`

`\\ \rm\Rrightarrow (x+8)(x-2)=0`

`\\ \rm\Rrightarrow x=-8\:and\:x=2`

Answer 2

(c) x = 2 and x = -8

Explanation:

The rules of logarithms let you rewrite this as a quadratic equation. That equation will have two (2) potential solutions. We know from the domain of the log function that any negative value of x will be an extraneous solution.

__

The rules of logarithms that apply are ...

`\log(a)+\log(b)=\log(ab)\qquad\text{all logarithms to the same base}\\\\\log_b(a)=c\ \Longleftrightarrow\ b^c=a\\\\`

__

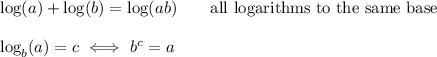
take antilogs

We can rewrite the equation so that only one logarithm is involved. Then we can take antilogs.

`\log_4(x)+\log_4(x+6)=2\\\\\log_4(x(x+6))=2\qquad\text{using the first rule}\\\\4^2=x(x+6)\qquad\text{using the second rule}`

__

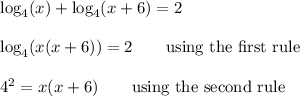
solve the quadratic

Adding (6/2)² = 9 to both sides will "complete the square."

16 +9 = x² +6x +9 . . . . . . . add 9

25 = (x +3)²

±√25 = x +3 = ±5 . . . . . take the square root(s)

x = -3 ±5 = {-8, +2}

The two potential solutions are x = 2 and x = -8.