Answer:
Equation of required line is:

Explanation:
We need to find equation for a line that's perpendicular to
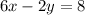 when it passes through (6,-5)
when it passes through (6,-5)
We will use the slope-intercept form:
 where m is slope and b is y-intercept.
where m is slope and b is y-intercept.
Finding Slope m:
When two lines are perpendicular there slopes are
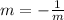
Finding slope of given equation first:
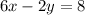
Writing in slope-intercept form:
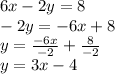
Comparing it with slope intercept form:
 , m= 3
, m= 3
So, slope of given equation is 3
Now, the slope of required equation will be
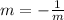
So, Slope m=-1/3
Finding y-intercept
Using slope m=-1/3 and point (6,-5) we can find y-intercept

So, y-intercept is b=-3
Now, finding the equation of required line having Slope m=-1/3 and y-intercept b= -3
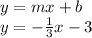
Writing in standard form:

So, equation of required line is:
