Given:
The line parallel to y = −x that passes through (7, 2.5).
To find:
The slope intercept form of the given line.
Solution:
Slope intercept form of the line is

where, m is slope and b is y-intercept.
On comparing y = −x with slope intercept form, we get
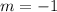
So, slope of y=-x is -1.
Slope of parallel lines are same. Thus, slope of required line is -1.
Required line passes through (7,2.5) with slope -1. So, the equation of line is



Add 2.5 on both sides.
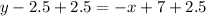

Therefore, the equation of the line that passes through (7, 2.5) is
 .
.