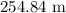Question

A rock is thrown with an initial vertical velocity 50 m/s at an angle of 40 degrees.

a. What is the horizontal component of the velocity?
b. What is the vertical component of the velocity?
c. What is the hang timel?
d. What is the peak height?
e. What is the range?

Answer 1

`38.3\ \text{m/s}`

`32.14\ \text{m/s}`

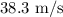
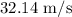
6.55 seconds

`52.65\ \text{m}`

`254.84\ \text{m}`

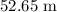
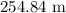
Step-by-step explanation:

u = Initial velocity of rock = 50 m/s

`\theta` = Angle of throw =
`40^(\circ)`

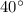
g = Acceleration due to gravity =
`9.81\ \text{m/s}^2`

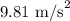
Horizontal component is given by

`u_x=u\cos\theta\\\Rightarrow u_x=50* \cos40^(\circ)\\\Rightarrow u_x=38.3\ \text{m/s}`

The horizontal component of the velocity is
`38.3\ \text{m/s}`

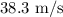
Vertical component is given by

`u_y=u\sin\theta\\\Rightarrow u_y=50* \sin40^(\circ)\\\Rightarrow u_y=32.14\ \text{m/s}`

The horizontal component of the velocity is
`32.14\ \text{m/s}`

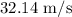
Time of flight is given by

`t=(2u\sin\theta)/(g)\\\Rightarrow t=(2* 50\sin40^(\circ))/(9.81)\\\Rightarrow t=6.55\ \text{s}`

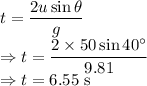
The hang time of the rock is 6.55 seconds

Maximum height is given by

`h=(u^2\sin^2\theta)/(2g)\\\Rightarrow h=(50^2\sin^240^(\circ))/(2* 9.81)\\\Rightarrow h=52.65\ \text{m}`

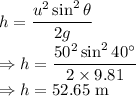
Maximum height is
`52.65\ \text{m}`

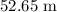
Range is given by

`d=(u^2\sin2\theta)/(g)\\\Rightarrow d=(50^2\sin(2*40)^(\circ))/(9.81)\\\Rightarrow d=254.84\ \text{m}`

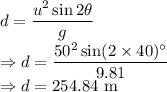
The range is
`254.84\ \text{m}`