Answer:
The zeros of the polynomial are -1 and 5
Explanation:
Quadratic Equation Solving
The standard representation of a quadratic equation is:

where a,b, and c are constants.
Solving with the quadratic formula:
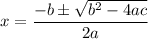
We have the following equation to solve:
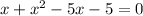
Before attempting to solve it, we must simplify the equation.
Collecting like terms and reordering:

Here: a=1, b=-4, c=-5
The discriminant of this quadratic equation is:
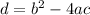
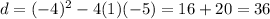
Given d is positive, the equation has two roots, and since d is a perfect square, both roots are rational.
Applying the formula:
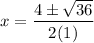
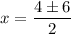
Dividing by 2:
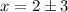
Separating both roots:
x = 2 + 3 = 5
x = 2 - 3 = -1
The zeros of the polynomial are -1 and 5