Question

In the diagram of AADC below, EB|| DC, ED=60, AB=12, and BC=72. What is the

length of AD?
A
12
E
B
60
72
D
С

Answer 1

The length of AD is 70

Explanation:

From the given figure

→ In triangle ADC

∵ EB // to DC

→ That means EB divides the sides AD and AC into parts proportional

∴ E divides AD into AE and ED

∴ B divides AC into AB and BC

∴
`(AE)/(ED)` =
`(AB)/(BC)`

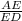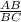
∵ ED = 60

∵ AB = 12

∵ BC = 72

→ Substitute them in the ratio above

∴
`(AE)/(60)` =
`(12)/(72)`

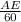→ By using cross multiplication

∵ AE × 72 = 12 × 60

∴ 72 AE = 720

→ Divide both sides by 72

∴ AE = 10

∵ AD = AE + ED

∴ AD = 10 + 60

∴ AD = 70

∴ The length of AD is 70