Question

The length of each side of an equilateral triangle is increased by 5 inches, so the perimeter is now 60 inches. Write and solve an equation to find the original length of each side of the equilateral triangle.

Answer 1

The original length of each side of the equilateral triangle = x =15 inches

Explanation:

Let Original length of side of equilateral triangle = x

If it is increased by 5 inches, the length will become = x+5

Since in equilateral triangle all the ides have same length so,

New Length of side 1 = x+5

New of side 2 = x+5

New Length of side 3 = x+5

Perimeter of triangle = 60 inches

We need to find the value of x

The formula used is:
`Perimeter=Length \ of \ side \ 1 \ + Length \ of \ side \ 2 \ + Length \ of \ side \ 3`

Putting values in formula and finding x

`Perimeter=Length \ of \ side \ 1 \ + Length \ of \ side \ 2 \ + Length \ of \ side \ 3\\60=x+5+x+5+x+5\\60=3x+15\\60-15=3x\\45=3x\\x=(45)/(3)\\x=15`

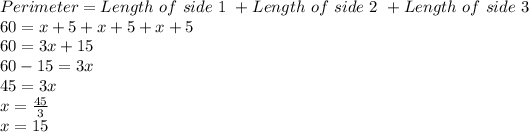
So, the original length of each side of the equilateral triangle = x =15 inches