Question

1. A soccer player runs directly up the field for 60 m before turning to the right at

an angle of 30° from his original direction and running an additional 20 m before
shooting a goal. What is the magnitude and direction of the player's total
displacement?

Answer 1

The magnitude of the displacement of the soccer player is approximately 77.96 m

The direction of the displacement of the soccer player to the right of the direction directly up the field is approximately 7.37°

Step-by-step explanation:

The given parameters are;

The distance the soccer player up the field = 60 m

The distance to the right the soccer player runs before shooting goal = 20 m

The direction to the right the soccer player turns from his original direction = 30°

Therefore, by resolution of the displacement, we have

d = 60·j + 20·cos(30°)·j + 20·sin(30°)·i

d = 60·j + 20·cos(30°)·j + 20·sin(30°)·i

d = 60·j + 20·(√3)/2·j + 10·i

d = 10·i + (60 + 10·√3)·j

The magnitude of the displacement of the soccer player
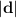`\left | \mathbf{d} \right |` = √(10² + (60 + 10·√3)²) ≈ 77.96 m

The direction of the displacement = tan⁻¹(10/(60 + 10·√3)) ≈ 7.37° to the right from the direction directly up the field