Explanation:
OPQ originally forms a sector, the formula for sector is
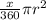
where x is the degrees of rotation between the two radii.
We know three sides length and is trying to find an angle between the radii so we can use law of cosines which states that
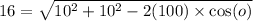
This isn't the standard formula, it's for this problem
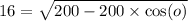
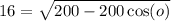
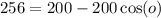
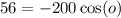
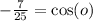
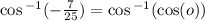
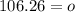
So we found our angle of rotation, which is 106.26
Now, we do the sector formula.
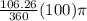
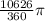
is the area of sector.
Now let find the area of triangle, we can use Heron formula,
The area of a triangle is
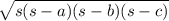
where s is the semi-perimeter.
To find s, add all the side lengths up, 10,10,16 and divide it by 2.
Which is
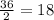
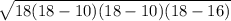
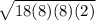
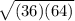
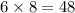
So our area of the triangle is 48. Now, to find the shaded area subtract the main area,( the sector of the circle) by the area of the triangle so we get
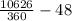
Which is an approximate or
44.73