Question

Helicopters rotor blades, could spin at high speed of 510 rpm. Find the angular displacement in radian for 3 hour(s) operation

Answer 1

The angular displacement of the blade is 576,871.2 radians

Step-by-step explanation:

Given;

angular speed of the Helicopters rotor blades, ω = 510 rpm (revolution per minute)

time of motion, t = 3 hours

The angular speed of the Helicopters rotor blades in radian per second is given as;

`\omega = (510 \ rev)/(mins) *(2 \pi \ rad)/(1 \ rev) *(1 \ min)/(60 \ s)\\\\\omega = 53.414 \ rad/s`

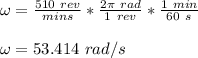
The angular displacement in radian is given as;

θ = ωt

where;

t is time in seconds

θ = (53.414)(3 x 60 x 60)\\

θ = 576,871.2 radians

Therefore, the angular displacement of the blade is 576,871.2 radians