Question

A jeweler wants to make 14 grams of an alloy that is precisely 75% gold.. The jeweler has alloys that are 25% gold, 50% gold, & 82% gold. Choose 2 different alloys that can be used to create one that is 75% gold. pls try to explain with a system of equations ; ;

Answer 1

Given that the jeweler has alloys that are 25% gold, 50% gold, and 82% gold.

As he wants to make 14 grams of an alloy by adding two different alloys that is precisely 75% gold, so one alloy must have a percentage of gold more than 75%.

One alloy is 82% gold and, the second can be chosen between 25% gold, 50% gold, so there are two cases.

Case 1: 82% gold + 50% gold

Let x grams of 82% gold and y grams of 50% gold added to make x+y=14 grams of 75% gold, so

75% of 14 = 82% of x + 50% of y

`\Rightarrow 75/100 * 14 = 82/100 * x + 50/100 * y \\\\`

`\Rightarrow 75/100 * 14 = 82/100 * x + 50/100 * (14-x)` [as x+y=14]

`\Rightarrow 75 * 14 = 82 * x + 50 * (14-x) \\\\\Rightarrow 75 * 14 = 82 * x + 50 *14-50* x \\\\\Rightarrow 75 * 14 = 32 * x + 50 *14 \\\\\Rightarrow 32 * x =75 * 14 - 50 *14 \\\\`

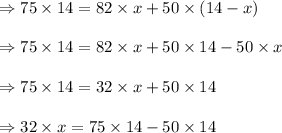
`\Rightarrow x =(25 * 14)/32=10.9375` grams

and
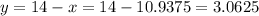`y = 14-x= 14-10.9375=3.0625` grams.

Hence, 10.9375 grams of 82% gold and 3.0625 grams of 50% gold added to make 14 grams of 75% gold.

Case 2: 82% gold + 25% gold

Let x grams of 82% gold and y grams of 25% gold added to make x+y=14 grams of 75% gold, so

75% of 14 = 82% of x + 25% of y

`\Rightarrow 75/100 * 14 = 82/100 * x + 25/100 * y \\\\\Rightarrow 75/100 * 14 = 82/100 * x + 25/100 * (14-x) \\\\ \Rightarrow 75 * 14 = 82 * x + 25 * (14-x) \\\\\Rightarrow 75 * 14 = 82 * x + 25 *14-25* x \\\\\Rightarrow 75 * 14 = 57 * x + 25 *14 \\\\\Rightarrow 57 * x =75 * 14 - 25 *14 \\\\`

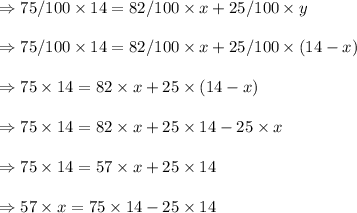
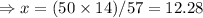`\Rightarrow x =(50 * 14)/57=12.28` grams

and
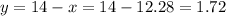`y = 14-x= 14-12.28=1.72` grams.

Hence, 12.28 grams of 82% gold and 1.72 grams of 50% gold added to make 14 grams of 75% gold.