Question

The sum of the ages of Peter and Paul is 24. Five years ago their respective ages were in the ratio 3:4. How old will they be in three years time?​

Answer 1

16 and 14 years old.

Explanation:

Let their ages be x and y years, then

x + y = 24 .......... (1)

Also from the given information

(x - 5)/ (y - 5) = 3/4

3y - 15 = 4x - 20

3y - 4x = -5

Multiplying equation (1) by 3:

3y + 3x = 72

Subtracting these last 2 equations:

-7x = -77

x = 11.

So y = 24 - 11 = 13.

So in 3 years time they will be 16 and 14.

Answer 2

Peter would be 14. Paul would be 16

Explanation:

Variable labels:

Peter = m, Paul = n

m + n = 24

(m - 5) : (n- 5) = 3:4

The ratio 3:4 in fraction form is
`(3)/(4)`

The ration of (m - 5) : (n- 5) in fraction form is
`(m - 5)/(n - 5)`

`(m-5)/(n-5) = (3)/(4)`

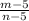
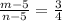
Cross multiply:

4(m - 5) = 3(n - 5)

4m - 20 = 3n - 15

4m = 3n + 5

Find the value of m first:

4m = 3n + 5

m =
`(3n + 5)/(4)`

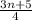
Now going back to the equation m + n = 24, substitute m with
`(3n + 5)/(4)` :

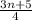m + n = 24

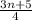`(3n + 5)/(4)` + n = 24

Solve for n:

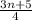`(3n + 5)/(4)` + n = 24

`(3n)/(4) + (5)/(4)` + n = 24

`(7n)/(4) +(5)/(4)` = 24

`(7n)/(4) = (91)/(4)`

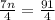
n = 13 (Paul's age)

Solve for m:

m + n = 24

m + 13 = 24

m = 11 (Peter's age)

After three years, Peter would be 11 + 3 = 14 years old and Paul would be 13 + 3 = 16 years old.