If you are in calculus, then you would apply the derivative to get
h(x) = x^2 - 2x + 3
h ' (x) = 2x - 2
The gradient, or slope of the tangent line, is negative when the derivative is negative.
So we need to solve h ' (x) < 0.
h ' (x) < 0
2x-2 < 0
2x < 2
x < 2/2
x < 1
Any x value smaller than x = 1 will lead to a negative gradient.
-----------------------------------
If you are not in calculus, then you can determine the vertex of the function
Note that y = x^2 - 2x + 3 is in the form y = ax^2 + bx + c with
a = 1
b = -2
c = 3
The x coordinate of the vertex is
h = -b/(2a)
h = -(-2)/(2*1)
h = 1
The x coordinate of the vertex is x = 1. Anything to the left of this will represent a negative gradient. This is because the given parabola opens upward (note how a = 1 is positive).
The graph goes downhill on the interval
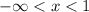 and it goes uphill on the interval
and it goes uphill on the interval
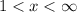 as shown below.
as shown below.