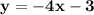Question

brooke found the equation of the line passing through the points (-7,25) and (-4,13) in slope-intercept form as follows​

Answer 1

The equation of the line passing through the points (-7,25) and (-4,13) in slope-intercept form is
`\mathbf{y=-4x-3}`

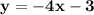
Explanation:

Equation of line passing through the points (-7,25) and (-4,13) in slope-intercept form.

The general equation of slope-intercept form is:
`y=mx+b`

First we need to find slope

The formula used for finding slope is:
`Slope=(y_2-y_1)/(x_2-x_1)`

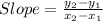
We are given:
`x_1=-7, y_1=25, x_2=-4, y_2=13`

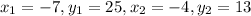
Putting values in formula and finding slope

`Slope=(y_2-y_1)/(x_2-x_1)\\Slope=(13-25)/(-4-(-7))\\Slope=(13-25)/(-4+7)\\Slope=(-12)/(3)\\Slope=-4`

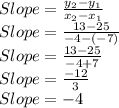
So, slope m= -4

Now finding y-intercept

Using slope m=-4 and point (-7,25) we can find y-intercept

`y=mx+b\\25=-4(-7)+b\\25=28+b\\b=25-28\\b=-3`

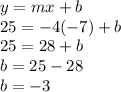
So, y-intercept b =-3

Now, the equation of required line having slope m=-4 and y-intercept b=-3 is:

`y=mx+b\\y=-4x-3`

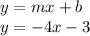
So, the equation of the line passing through the points (-7,25) and (-4,13) in slope-intercept form is
`\mathbf{y=-4x-3}`