Question

Consider the following graph which represents the solutions to a system of inequalities. Which of the following systems of inequalities does the graph represent?​

Answer 1

The system of inequalities is -2x + y > 0 -x + y ≥ 0

Explanation:

i did it

Answer 2

The system of inequalities is -2x + y > 0 -x + y ≥ 0

Explanation:

The form of the equation of a line is y = m x + b, where

• m is the slope

• b is the y-intercept

The line passes through the origin, then

• The value of b = 0

• The form of the equation is y = m x

Let us look at the graph to find the correct answer

∵ One of the lines are solid and the other is dashed

∵ The shaded area is over the two lines

∴ The signs of the two inequalities are ≥ and >

∵ The two lines pass through the origin

→ That means the y-intercepts are 0

∴ The form of the inequalities are y ≥ m
`_(1)` x and y > m
`_(2)`

There are only two answers that have these forms, so we must find the slope of each line

∵ m =
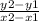`(y2-y1)/(x2-x1)` , (x1, y1) and (x2, y2) are two points on the line

∵ The solid line passes through points (0, 0) and (2, 2)

∴ m
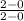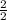`_(1)` =
`(2-0)/(2-0)` =
`(2)/(2)` = 1

→ Substitute it in the form of the equation

∴ y ≥ 1(x)

∴ y ≥ x

→ Subtract x from both sides

∴ -x + y ≥ 0

∵ The dashed line passes through points (0, 0) and (1, 2)

∴ m
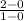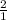`_(2)` =
`(2-0)/(1-0)` =
`(2)/(1)` = 2

→ Substitute it in the form of the equation

∴ y > 2(x)

∴ y > 2x

→ Subtract 2x from both sides

∴ -2x + y > 0

The system of inequalities is -2x + y > 0 -x + y ≥ 0