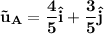Answer:
Step-by-step explanation:
Unit Vector
The unit vector associated with a given vector
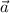 is another vector pointing in the same direction of
is another vector pointing in the same direction of
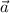 and with magnitude 1.
and with magnitude 1.
The unit vector can be calculated as follows:
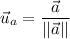
Where
 is the magnitude of the vector.
is the magnitude of the vector.
If
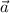 is given as:
is given as:
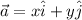
The magnitude of the vector is:
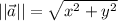
We have:
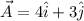

Thus the unit vector is:
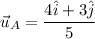
Simplifying: